Let's look once more at the equation
$$E=\frac{m}{\sqrt{1-v^2}}$$
This looks an awful lot like the equation for time dilation. $E$ is the mass as measured by someone who sees the object moving at $v$ whereas $m$ is the mass as measured by someone who sees the object at rest, e.g. by the object itself.
Similarly, we have the equation $p=vE$, which looks an awful lot like the equation $x=vt$. It therefore makes sense to wonder how far this analogy goes. We could start with analysing the invariant.
Even if I measure the mass of a 1kg rock as 10kg because of my reference frame, I know that if I brought the bag to rest, I would measure it as 1kg. Much like I can tell people's biological age or look at their clocks to determine their proper time, I can look at the moving thing's mass balance and determine its proper mass $m$.
If we just wanted $m$ in terms of the "co-ordinates" $E$ and $p$,
$$m = E\sqrt {1 - {v^2}} = \sqrt {{E^2} - {v^2}{E^2}} = \sqrt {{E^2} - {p^2}}$$
$${m^2} = {E^2} - {p^2}$$
Or in 4 dimensions,
$${m^2} = {E^2} - p_x^2 - p_y^2 - p_z^2$$
We call $m$ the "proper mass". In general, "proper" means "as measured in the rest frame" -- proper time, proper length, proper mass, whatever. This equation is also useful because unlike the previous thing, this also works when $v=1$ (i.e. for light), and reduces to $E=pc$.
But this looks an awful lot like the spacetime interval.
That's not all. Consider an object with mass $E$, momentum $p$ and velocity $w=p/E$ in our reference frame $O$. Now boost to a reference frame $O'$ with relative velocity $v$ to $O$. Then the velocity of the object has transformed from $w$ to $\frac{{w - v}}{{1 - wv}}$. So
$$\begin{array}{c}E' = \frac{m}{{\sqrt {1 - {{\left( {\frac{{w - v}}{{1 - wv}}} \right)}^2}} }}\\ = \frac{{m(1 - vw)}}{{\sqrt {{{(1 - wv)}^2} - {{(w - v)}^2}} }}\\ = \frac{{m(1 - vw)}}{{\sqrt {(1 - {w^2})(1 - {v^2})} }}\\ = \gamma (v)\left( {1 - vw} \right)\gamma (w)m\\ = \gamma \left( {1 - vw} \right)E\\ = \gamma (E - vwE)\\E' = \gamma (E - vp)\end{array}$$
And
$$\begin{array}{c}p' = \frac{{m\left( {\frac{{w - v}}{{1 - wv}}} \right)}}{{\sqrt {1 - {{\left( {\frac{{w - v}}{{1 - wv}}} \right)}^2}} }}\\ = \left( {\frac{{w - v}}{{1 - wv}}} \right)E'\\ = \left( {\frac{{w - v}}{{1 - wv}}} \right)\gamma \left( {1 - wv} \right)E\\ = \gamma (wE - vE)\\p' = \gamma (p - vE)\end{array}$$
Or alternatively
$$\left[ \begin{array}{l}{E'}\\{p'}\end{array} \right] = \gamma \left[ {\begin{array}{*{20}{c}}1&{ - v}\\{ - v}&1\end{array}} \right]\left[ \begin{array}{l}E\\p\end{array} \right]$$
In 4 dimensions,
$$\left[ \begin{array}{l}{E'}\\{{p'}_x}\\{{p'}_y}\\{{p'}_z}\end{array} \right] = \left[ {\begin{array}{*{20}{c}}1&{ - v}&{}&{}\\{ - v}&1&{}&{}\\{}&{}&1&{}\\{}&{}&{}&1\end{array}} \right]\left[ \begin{array}{l}E\\{p_x}\\{p_y}\\{p_z}\end{array} \right]$$
Which is precisely the transformation for time and position.
We call vectors that transform like this spacetime vectors or four-vectors. Four-vectors all share the same algebraic properties -- they transform in the same way, they follow vector addition, their norms and in general their dot products are invariant, etc. -- but not necessarily other properties. E.g. energy and momentum have conservation laws, but position and time do not.
The norm of a spacetime vector is taken as:
$${\left| {\left[ {\begin{array}{*{20}{c}}{{q_0}}\\{{q_1}}\\{{q_2}}\\{{q_3}}\end{array}} \right]} \right|^2} = q_0^2 - q_1^2 - q_2^2 - q_3^2$$
Which is distinct from the Euclidean norm, once again telling us that the geometry of spacetime is not Euclidean.
Four-vectors are perhaps the most beautiful example of the symmetry between space and time. They essentially allow you to replace ordinary pre-relativistic vectors like momentum with vectors that also have a time component alongside three spatial components, because the world is 4-dimensional. You just need to find a quantity that behaves with the vector like time behaves with position -- i.e. you need to show the two quantities transform between each other in a Lorentz transformation sort of way.
You end up with truly mind-boggling results -- we already saw that mass is the time-component of momentum, which explains why mass produces inertia -- an object with mass already devotes a lot of its momentum to moving forward in time, so the more the mass, the more of this momentum you need to transform into the spatial direction. This is really what is meant by the transformation law $p'=\gamma(p-vE)$ for mass $E$, generalising the Galilean $p'=p-vE$ (change $E$ to $M$ if that makes you happy). It also explains why massless (meaning zero rest mass) things can move at the speed of light.
Other such four-vectors include:
- Four-force (time-component: $dE/dt$)
- Four-current (time-component: charge density, space-component: current density)
- Electromagnetic four-potential
Other quantities, like the electric and magnetic fields, even though they follow similar invariants (in the electromagnetic field example $E^2-B^2$), do not combine to form four-vectors, but instead objects called "tensors", which we will eventually talk about.
Note that during this transformation (giving something momentum), both mass and momentum increase. Similarly, time dilates when you move something around. This is again because $E^2-p^2$, not $E^2+p^2$ is invariant. The latter would correspond to a circular rotation, with invariant circles, whereas the former corresponds to a skew (a "hyperbolic rotation"), with invariant hyperbolae.
Rapidity and hyperbolic rotations
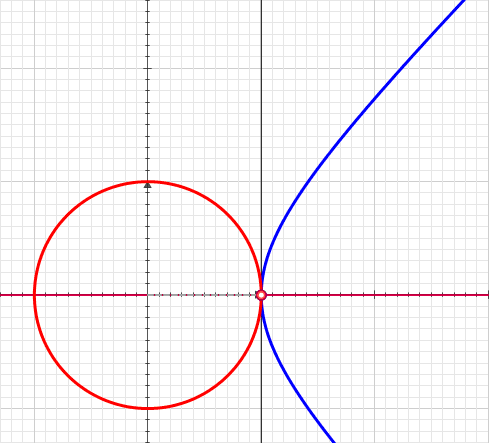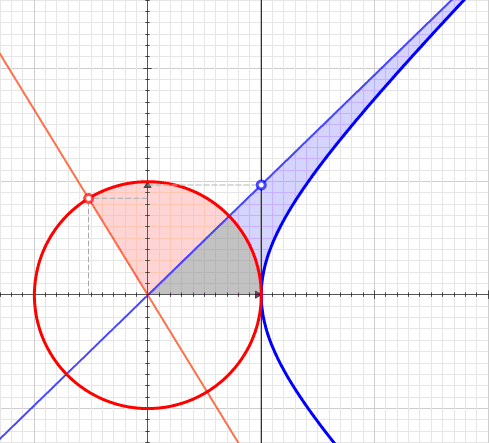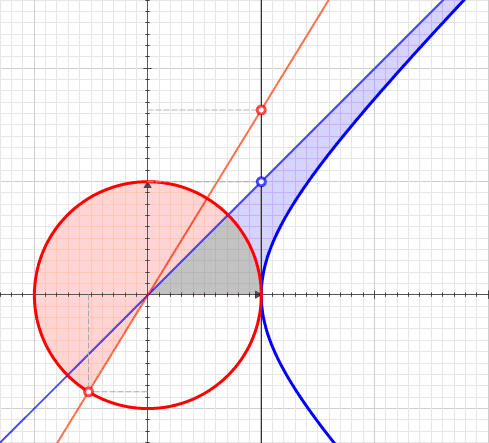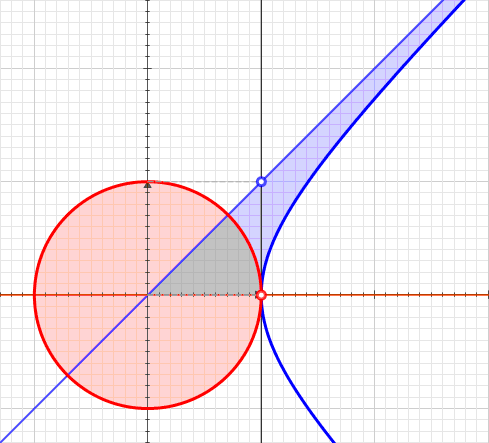
Points $(\cos\theta,\sin\theta)$, $(1,\tan\theta)$, $(\cosh\xi,\sinh\xi)$ and $(1,\tanh\xi)$ plotted for varying $\theta$ and $\xi$. While only $\theta$ can be interpreted as an angle too, both $\theta$ and $\xi$ can be interpreted as areas.
This will be a bit of a DIY section, with some guidance.
QUESTION 1
(a) Consider the equation $v' = \frac{{v - w}}{{1 - vw}}$. What trigonometric identity does this remind you of? Could you resolve the differences somehow? (Hint: $v=\tanh\xi$)
(b) Prove that the Lorentz transformations can be written as
$$\begin{array}{l}t' = t\cosh \xi - x\sinh \xi \\x' = x\cosh \xi - t\sinh \xi \end{array}$$
(c) Use the hyperbolic analog of angle-addition formulae to show that this is equivalent to, where $\phi=\mathrm{artanh}(x/t)$ is the rapidity of the point $(t,x)$ in the original reference frame.
$$\begin{array}{l}t' = s\cosh (\phi - \xi )\\x' = s\sinh (\phi - \xi )\end{array}$$
(d) The above result means that rapidity transforms as $\phi ' = \phi - \xi $ (which is itself nice, because it tells you that velocity at low speeds is approximately equal to rapidity by a factor of $c$) and $(t,x) = (s\sinh \phi ,s\cosh \phi )$. Relate the former to the idea of invariant hyperbolae and the interpretation of rapidity as an area (hint, hint: area sweeped out by a conic section... Kepler).
QUESTION 2
(a) Results 1(b) and 1(c) are very similar to the effect of rotations on co-ordinate transformations. Here the linear transformations are skews, not rotations, which is why the formulae are different. Draw as many analogs as you can between rotations and skews in linear algebra. Refer to Article 1103-006. Think about the rotational transformation matrix, etc.
(b) Consider (a) directly in the context of special relativity. Pretending that Lorentz boosts are simply rotations (which would imply a metric signature (+,+,+,+) and treat time exactly like space), explain transformations between time and position, etc. Relate this to the actual, skew-y Lorentz transformations. Describe how relativity would behave in this theory.
(c) Write as many relativistic things as you can in the language of rapidity -- the Lorentz factor, the Doppler factor, components of a four-vector (how do $E$ and $p$ look in terms of rapidity), etc.
(d) Graph the hyperbolic functions and explain why the graphs make the results in 2(b) make sense.
(e) How does rapidity interpretation make certain things, like $c$ being the maximum speed, natural?
QUESTION 3
(a) Consider once again the transformation $\phi ' = \phi - \xi $. What does this tell you about the relative rapidity $\Delta\phi$? Is this invariant, i.e. do all observers agree on what the relative rapidity between two objects is, like observers did on relative velocity in Galilean relativity?
(b) Explain why it would be foolish to expect the quantity $\arctan{v}$, the Euclidean angle (as opposed to rapidity, which we may call the "Minkowskian angle"), to have any physical significance. Think about the quantity $r\arctan{v}$ where $r^2=\Delta t^2+\Delta x^2$ (no minus sign).
It's therefore reasonable to define the dot product on spacetime as $\vec a \cdot \vec b = |\vec a||\vec b|\cosh \Delta \phi $ where $\Delta\phi$ is the relative rapidity/Minkowskian angle/difference in rapidity. This expression implies that $|\vec a|^2=\vec a\cdot\vec a$is manifestly (i.e. obviously) Lorentz invariant, since both norms and relative rapidity are invariant.
(c) Translate this out of rapidity language, i.e. into a language where rapidity is not used as a parameterisation. You should get $a_0b_0-a_1b_1$ (where 0 and 1 are the temporal and spatial components respectively) in two dimensions.
The fact that this modified dot product is invariant under a skew is analogous to how the standard dot product is invariant under rotations ("complex skews"). Indeed, it turns out see that the 4-dimensional Minkowski dot product
$${a_0}{b_0} - {a_1}{b_1} - {a_2}{b_2} - {a_3}{b_3}$$
Is invariant under skews (between the time axis and some other axis) as well as spatial rotations (and all combinations thereof -- i.e. a general Lorentz transformation), as it contains both a "skew-y" part and a "standard dot product-y" part.
Some interesting things regarding 2(b):
A circular Lorentz transformation would transform position and time something similar to this:
$$\begin{array}{l}x' = \eta (x - vt)\\t' = \eta (t + vx)\end{array}$$
One can also talk about transforming the positive and negative sides of the axes separately.
$$\begin{array}{l}x' = \eta (x - vt)\\t' = \eta (t + vx)\\ - x' = \eta ( - x - v( - t))\\ - t' = \eta ( - t - v( - x))\end{array}$$
No comments:
Post a Comment