Jevon's theorem and economic intuitions

Recent discourse surrounding Jevon’s paradox provides an opportunity to flesh out the precise intuitions behind marginalist economics and how concepts like “elasticity” arise naturally from agent/graph-based first principles.

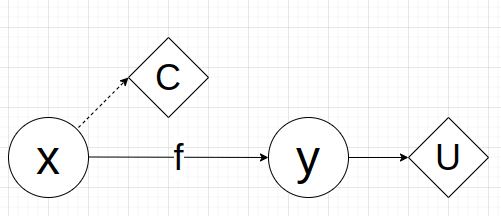
Say you have resource $x$ (e.g. compute) with cost $C(x)$ that produces good y (e.g. AI), with utility function $U(x)$ via production function $y = f(x)$.

The market maximizes $U(f(x)) - C(x)$ — note that the derivatives $U'(f(x))f'(x)$ and $C'(x)$ are exactly the MB and MC curve for $x$, so this is just intersecting demand and supply for $x$.

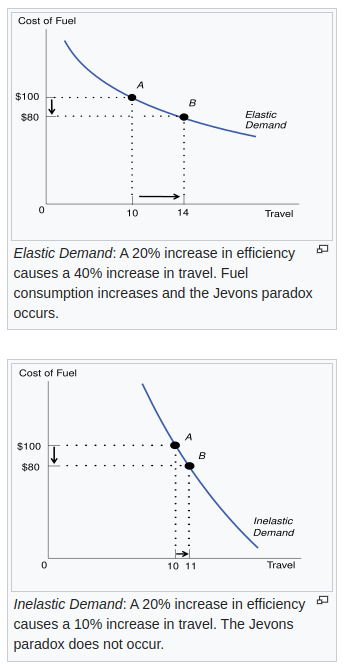
Here is the explanation on Wikipedia and other places … this is not actually wrong (as we’ll see), but if you think about it, it really looks wrong!

Contrary to what the above figure appears to show, an efficiency improvement is *not* a shift of $C(x)$ at all! It is a shift of $f(x)$, e.g. from the function $f(x)=x$ to $f(x)=2x$.

Jevon's paradox is then quite intuitive — you're increasing how valuable $x$ is ($U(f(x))$ increases) when $f’(x)$, so of course the consumption of $x$ will increase. Its MB curve is literally shifting to the right!

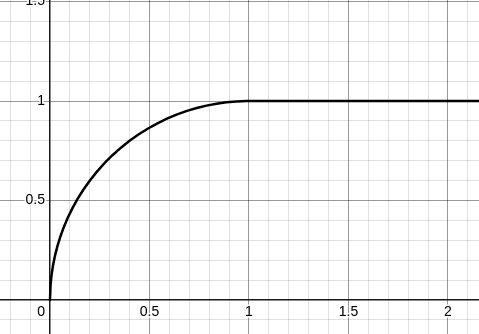
*Unless* if y is something you only have "need not greed™" for, i.e. if $U(y)$ diminishes really quickly after a point. If the world only needs 200kg of AI, and has no use for anymore, then you can now make that with much less compute and you’re done.

So e.g. with $U(y)=\log(1+y)$, $C(x)=x^2$ you see Jevon’s paradox. [Pictured below](https://www.desmos.com/calculator/a6rtjhdqfk): $U(ax)-C(x)$ as $a$ increases — you can see that the argmax increases.

But with this $U(y)$:

We have the following behaviour:

We can generalize this: in general, Jevon’s paradox holds—

$\iff$ $\arg\max U(ax) - C(x)$ increases as $a$ increases

$\iff$ $U(ax) - C(x)$ rises faster with $a$ for larger $x$ than for smaller $x$

$\iff$ $\frac{\partial}{\partial a} {\left[U(ax)-C(x)\right]}$ increases with $x$

$\iff$ $xU'(ax)$ increases with $x$.

$\iff$ $U'(ax) + axU''(ax) > 0$

$\iff$ $MB(y) + yMB'(y) > 0$

$\iff$ $MB(y)/(yMB'(y)) < -1$

$\iff$ $PED(y) < -1$